有限时间控制具有良好的动态性能、较高的稳态精度以及有限时间收敛的特性,在机器人控制上得到了广泛的应用 [ 9- 10] . 但是,有限时间控制依赖系统初始条件,实际系统的初始值可能无法提前获得,从而无法准确计算出系统的收敛时间. 固定时间控制方法的收敛时间上界与初始条件无关,已成为新的研究热点 [ 11] . Zhang等 [ 12] 提出基于扩张状态观测器的固定时间输出反馈滑模跟踪控制方法,用以实现系统跟踪误差在固定时间内稳定到原点. Zuo [ 13] 针对存在匹配扰动的二阶非线性系统提出固定时间终端滑模控制策略. Ni等 [ 14] 提出固定时间稳定系统,并基于此系统推导出固定时间非奇异快速终端滑模控制器. 由于变指数幂次的存在,该收敛系统比常值幂次项的系统具有更快的收敛速度.
本研究考虑存在扰动情况下的双臂空间机器人轨迹跟踪问题,提出固定时间非奇异快速终端滑模控制策略. 将固定时间滑模面与固定时间趋近律结合,设计固定时间非奇异快速终端滑模控制器,实现基座以及机械臂关节在固定时间内跟踪上期望轨迹.
1. 问题描述与预备知识
1.1. 双臂空间机器人动力学模型
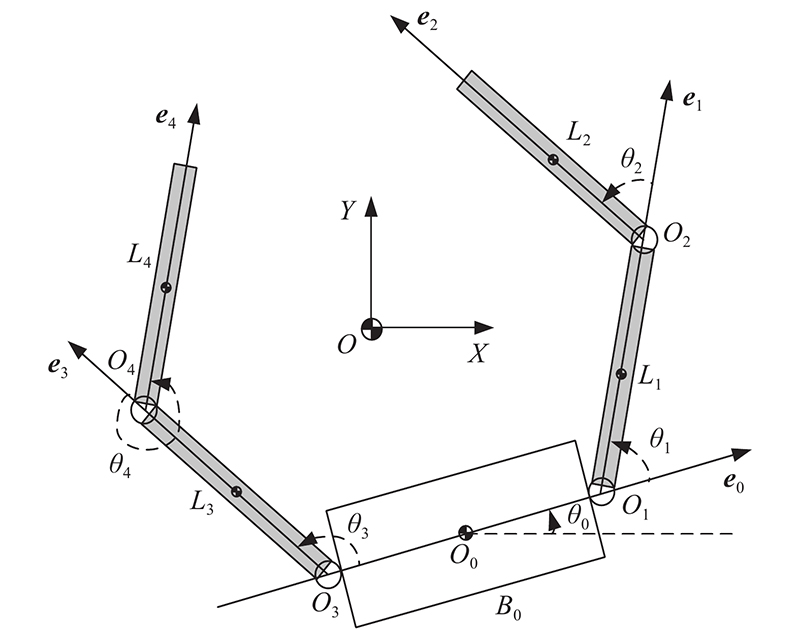
如 图1所示,以平面双臂空间机器人为例,建立其动力学模型.该结构包含中心基座
图 1
双臂空间机器人系统的动力学方程 [ 15] :
式中:
式中:
假设1 在动力学方程式(1)、(2)中,惯量矩阵
1.2. 控制目标
针对式(2),在假设成立的条件下,设计固定时间非奇异快速终端滑模控制律
1.3. 预备知识
引理1 考虑非线性系统
引理2 考虑如下的非线性系统
式中:
证明 式(4)可被分解为分段函数:
变换式(5),当
求解式(6)得到系统的收敛时间上界为
引理2中系统固定时间收敛特性得证.
2. 控制器设计
2.1. 固定时间非奇异快速终端滑模控制器设计
为了方便控制器设计,将式(2)改写为如下的状态方程形式:
式中:
将基座期望姿态角以及各关节给定的期望轨迹记为
式中:
式中:
令
式中:
为了提高滑模控制趋近阶段的收敛速度,削弱滑模控制固有的抖振问题,设计趋近律:
式中:
结合式(8)、(13)、(14),本研究设计的固定时间非奇异快速终端滑模律为
式中:
2.2. 收敛性证明
定理 考虑双臂空间机器人系统,采用本研究设计的固定时间非奇异快速终端滑模面. 在固定时间控制律的作用下,滑模面
证明 构造李雅普诺夫函数:
对(16)求导,同时结合式(8)、(11)、(12)可以得到
将式(13)、(15)代入式(17),有
当
当
根据李雅普诺夫稳定性定理和引理2可知,系统跟踪误差在固定时间
此时,由式(9)可知
当
此时,
当
因此
综上,系统跟踪误差在固定时间内收敛且收敛时间满足
3. 仿真与分析
表 1 双臂空间机器人质量特性参数
Tab.1
| 分体 | | | |
| | 40 | 1.5 | 34.17 |
| | 2 | 3 | 1.50 |
| | 1 | 3 | 0.75 |
| | 2 | 3 | 1.50 |
| | 1 | 3 | 0.75 |
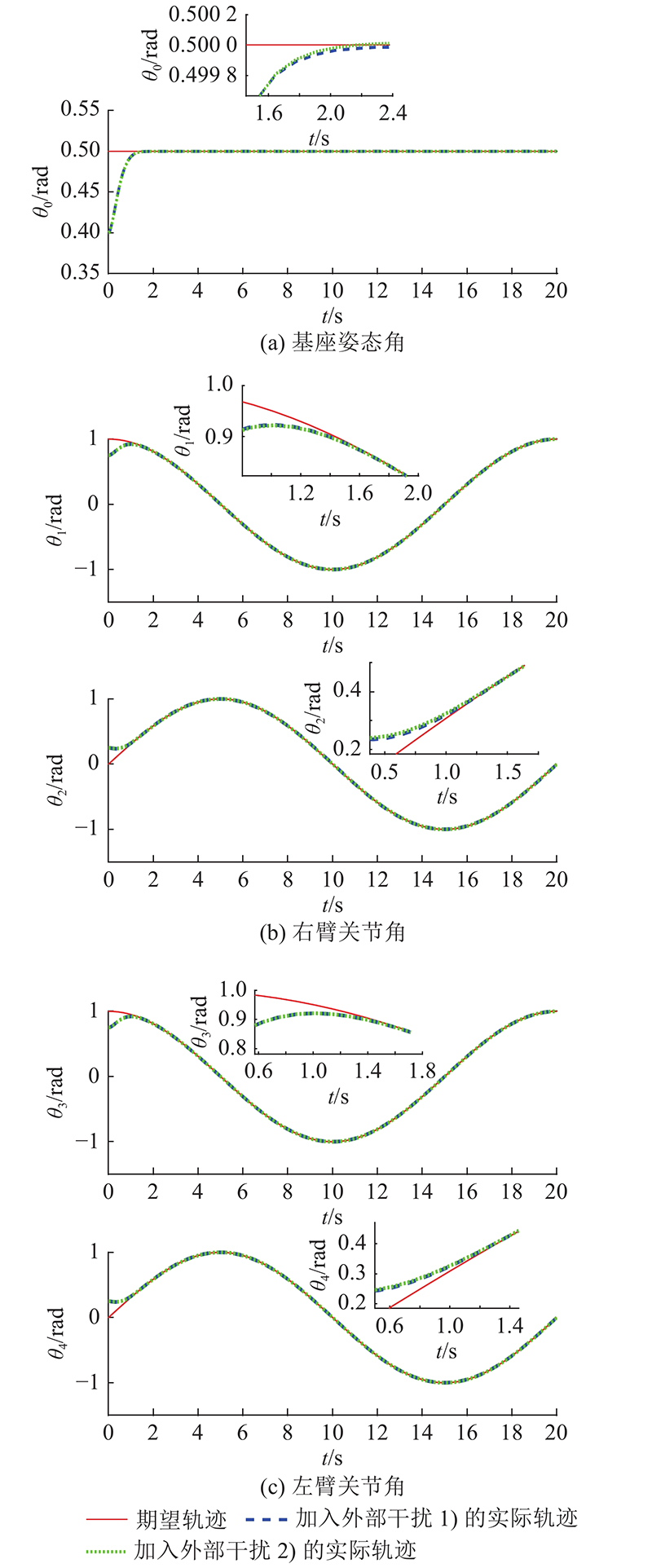
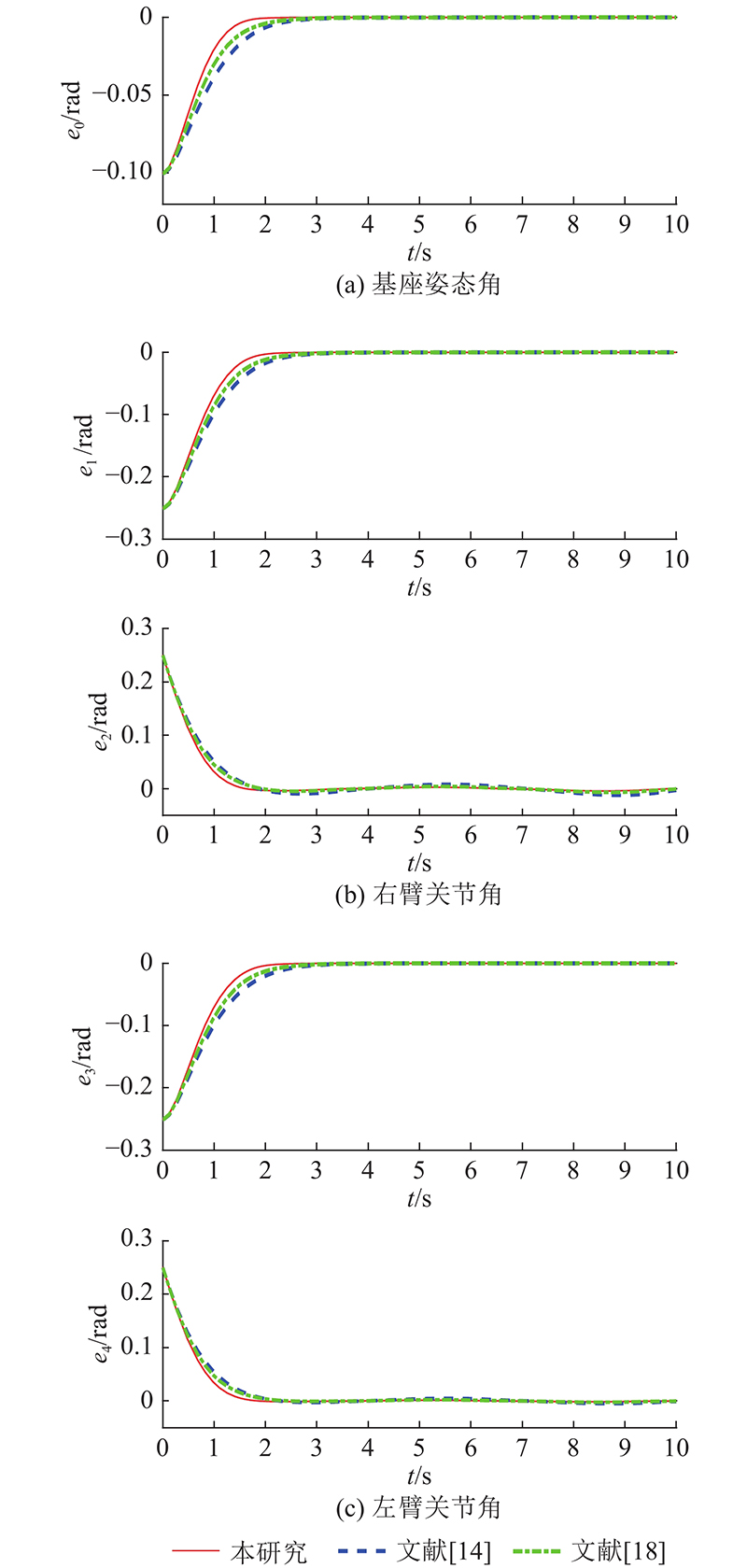
仿真1 基座姿态角以及机械臂关节角的初始状态为
图 2
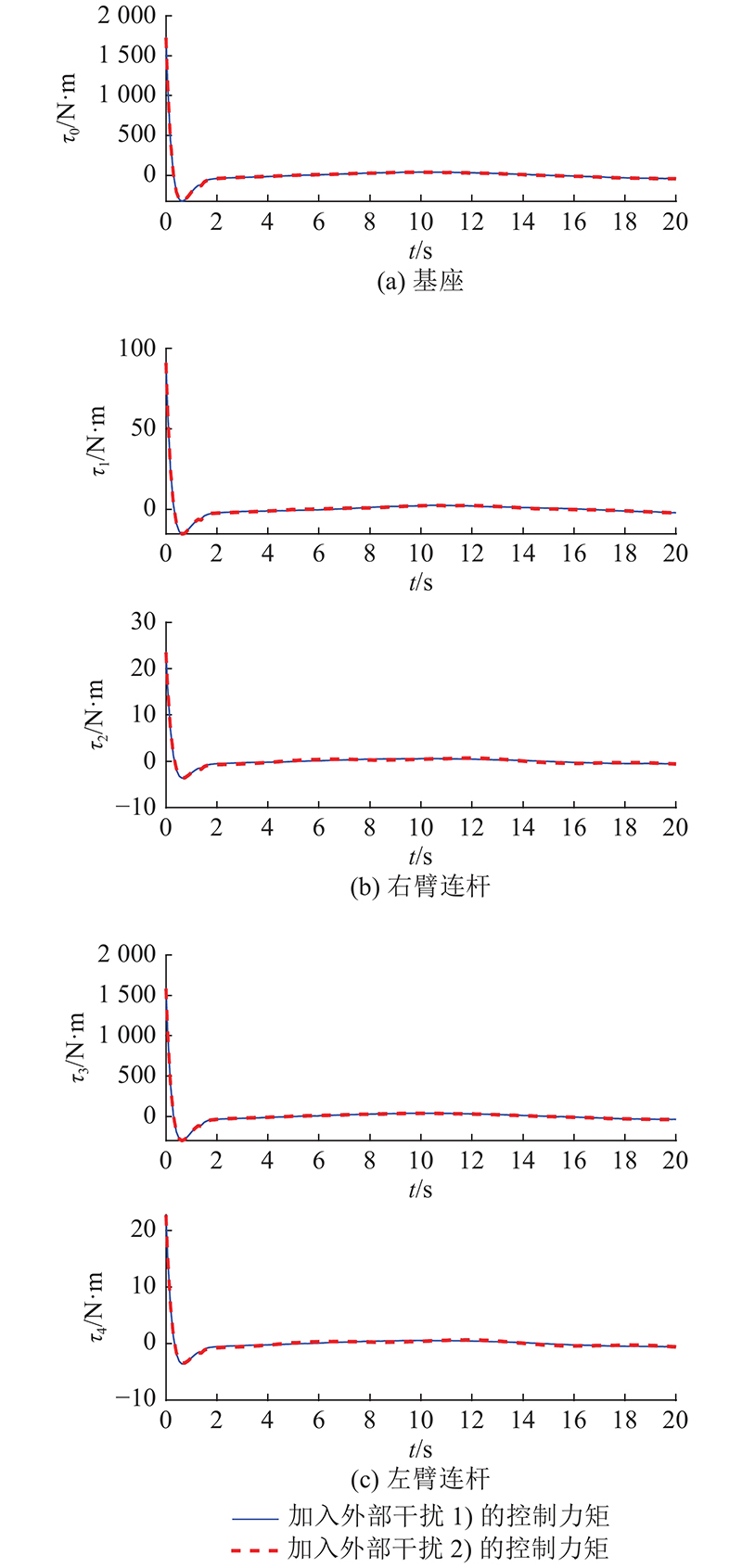
图 3
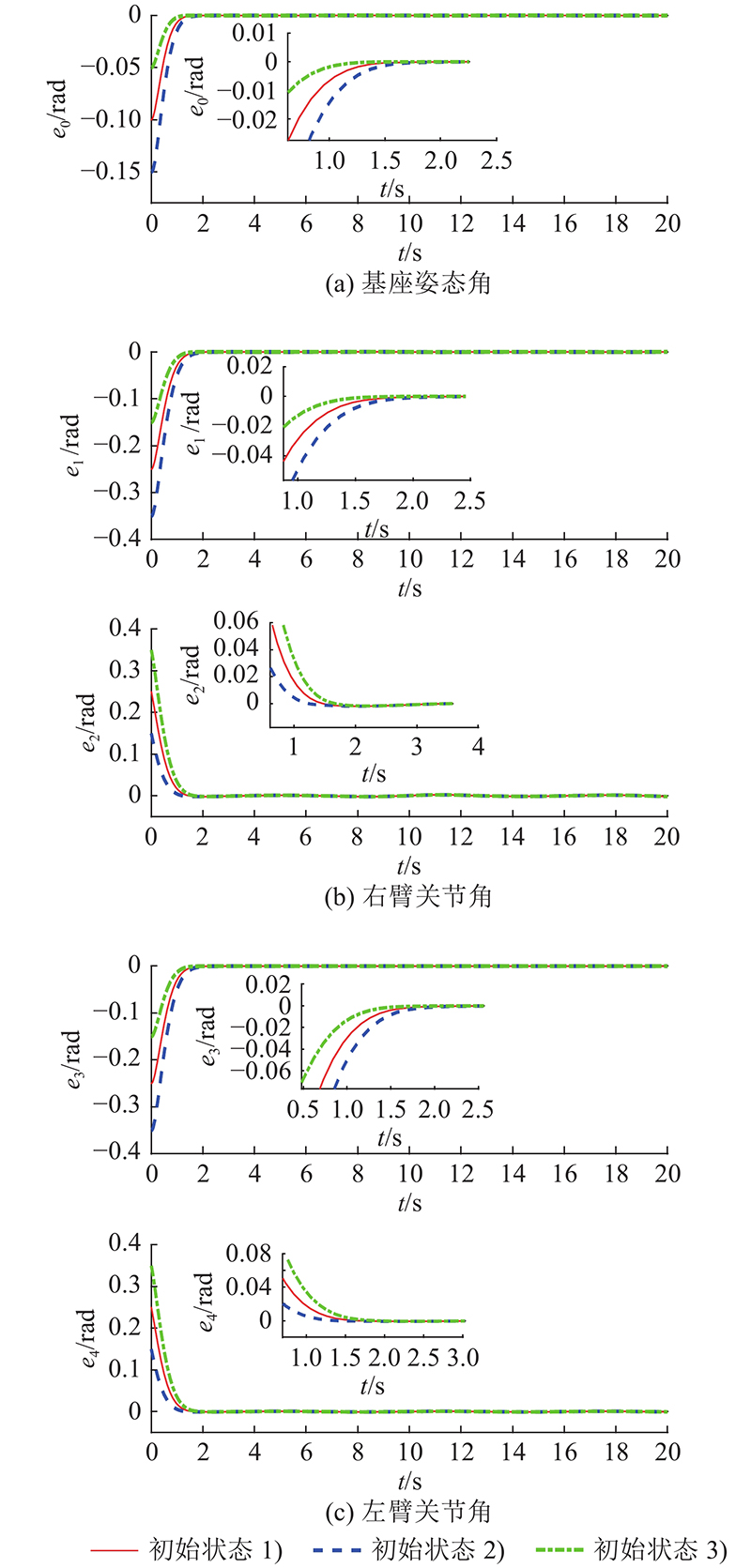
仿真2 为了验证本研究所提固定时间控制算法的跟踪效果不受系统初始状态的影响,定义仿真1中基座姿态角以及机械臂关节角的初始状态为初始状态1). 再设置初始状态2)为
初始状态3)为
控制律参数保持不变,基座及双臂关节轨迹跟踪结果如 图4所示. 图中,
图 4
图 5
图 5 不同固定时间控制律下跟踪误差收敛时间对比
Fig.5 Convergence time under different fixed-time controllers
4. 结 论
(1)针对扰动情况下双臂空间机器人轨迹跟踪问题,提出固定时间非奇异快速终端滑模控制策略,实现基座姿态角和机械臂关节角在固定时间内跟踪期望轨迹,证明收敛时间与系统初始值无关.
(2)在滑模面设计部分,利用固定时间理论,设计非奇异快速终端滑模面. 该滑模面不但具有固定时间收敛特性,而且比现有固定时间滑模面的收敛速度更快.
(3)提出快速终端滑模形式的趋近律,不但提高了系统趋近阶段收敛速度,而且削弱了控制力矩抖振现象.
(4)本研究局限于空间机器人的关节轨迹跟踪控制,在后续的研究工作中,将关注双臂空间机器人末端轨迹的跟踪控制.
参考文献
Trajectory planning of free-floating space robot using particle swarm optimization (PSO)
[J].DOI:10.1016/j.actaastro.2015.03.008 [本文引用: 1]
保持基座稳定的双臂空间机器人轨迹规划研究
[J].DOI:10.1016/S1874-1029(13)60008-7 [本文引用: 1]
Study on trajectory planning of dual-arm space robot keeping the base stabilized
[J].DOI:10.1016/S1874-1029(13)60008-7 [本文引用: 1]
Trajectory planning of dual-arm space robots for target capturing and base manoeuvring
[J].DOI:10.1016/j.actaastro.2019.08.004 [本文引用: 1]
空间双臂机器人抓捕翻滚目标后的鲁棒稳定控制
[J].DOI:10.6052/0459-1879-20-449 [本文引用: 1]
A roubst stabilization control for dual-arm space robot capturing tumbling target
[J].DOI:10.6052/0459-1879-20-449 [本文引用: 1]
配置柔顺机构空间机器人双臂捕获卫星操作力学模拟及基于神经网络的全阶滑模避撞柔顺控制
[J].DOI:10.6052/0459-1879-18-407 [本文引用: 1]
Mechanical simulation and full order sliding mode collision avoidance compliant control based on neural network of dual-arm space robot with compliant mechanism capturing satellite
[J].DOI:10.6052/0459-1879-18-407 [本文引用: 1]
Coordinated control of a dual-arm space robot: novel models and simulations for robotic control methods
[J].DOI:10.1109/MRA.2018.2864717 [本文引用: 1]
Multi-objective configuration optimization for coordinated capture of dual-arm space robot
[J].DOI:10.1016/j.actaastro.2019.11.002 [本文引用: 1]
Finite-time H ∞ control for high-precision tracking in robotic manipulators using backstepping control
[J].DOI:10.1109/TIE.2016.2583998 [本文引用: 1]
Space manipulator trajectory tracking based on recursive decentralized finite-time control
[J].DOI:10.1016/j.ast.2020.105870 [本文引用: 1]
Nonlinear feedback design for fixed-time stabilization of linear control systems
[J].DOI:10.1109/TAC.2011.2179869 [本文引用: 1]
Fixed-time output feedback sliding mode tracking control of marine surface vessels under actuator faults with disturbance cancellation
[J].DOI:10.1016/j.apor.2020.102378 [本文引用: 1]
Non-singular fixed-time terminal sliding mode control of non-linear systems
[J].DOI:10.1049/iet-cta.2014.0202 [本文引用: 3]
Fast fixed-time nonsingular terminal sliding mode control and its application to chaos suppression in power system
[J].DOI:10.1109/TCSII.2016.2551539 [本文引用: 4]
漂浮基双臂空间机器人系统协调运动的模糊小波神经网络控制
[J].
Fuzzy wavelet neural network control for coordinated motion of a free-floating dual-arm space robot
[J].
Fixed-time attitude control for rigid spacecraft with actuator saturation and faults
[J].DOI:10.1109/TCST.2016.2519838 [本文引用: 1]
Adaptive super-twisting nonsingular fast terminal sliding mode control for cable-driven manipulators using time-delay estimation
[J].DOI:10.1016/j.advengsoft.2018.11.006 [本文引用: 1]