浙江大学学报(工学版)

浙江大学学报(工学版), 2021, 55(1): 189-194 doi: 10.3785/j.issn.1008-973X.2021.01.022

电气工程

磷酸铁锂电池内阻分量快速检测方法

潘斌,, 董栋, 钱东培, 钮树强, 刘双宇, 姜银珠,

1. 浙江大学 材料科学与工程学院，浙江 杭州 310058

2. 浙江华云信息科技有限公司，浙江 杭州 310008

Quick identification of internal resistance components for lithium ion battery with LiFePO4 cathode

PAN Bin,, DONG Dong, QIAN Dong-pei, NIU Shu-qiang, LIU Shuang-yu, JIANG Yin-zhu,

1. School of Materials Science and Engineering, Zhejiang University, Hangzhou 310058, China

2. Zhejiang Huayun Imformation Technology Limited Company, Hangzhou 310008, China

通讯作者: 姜银珠，男，教授. orcid.org/0000-0003-0639-2562. E-mail： yzjiang@zju.edu.cn

收稿日期: 2020-06-10  

Received: 2020-06-10  

作者简介 About authors

潘斌（1989—），男，博士，从事电池检测技术的研究.orcid.org/0000-0002-0467-4681.E-mail：panbinsp@zju.edu.cn , E-mail：panbinsp@zju.edu.cn

摘要

为了实现锂离子电池（LIB）内阻分量快速检测，提出通过直流内阻（DCR）测试及交流内阻测试，辨识各内阻分量的方法. 以磷酸铁锂电池为研究对象，分别采用Bulter-Volmer方程和二阶等效电路模型，模拟研究表征界面电荷转移、浓差极化过程等效电路的时间常数. 由于电荷转移速度足够快，采用直流脉冲测试获得的瞬时响应内阻通常由欧姆内阻和电化学极化内阻组成，结合交流内阻测试仪内阻检测结果，可以计算得到电化学极化内阻分量. 实验结果显示，该内阻分量测试方法不仅操作简便且具有较高的可靠性，电化学极化内阻辨识结果与电化学阻抗测试结果的最小误差小于5%.

关键词： 锂离子电池（LIB） ; 健康状态 ; 内阻 ; 等效电路 ; 时间常数

Abstract

A resistance identification method was proposed by measuring direct current resistance (DCR) and alternating current (AC) resistance in order to quickly test internal resistance components of lithium ion battery (LIB). The characteristics and time constants of each resistance component were analyzed in a case study of LiFePO4 (LFP) battery based on Bulter-Volmer equation and the second order equivalent circuit model. Since the charge transfer process is fast enough, the resistance corresponding to the instantaneous voltage change in DCR test generally includes Ohmic resistance and charge transfer resistance. Then the charge transfer resistance can be distinguished combined with AC internal resistance test results. Results show that the proposed method with simple operations is effective and reliable. The minimum error of the charge transfer resistance is no larger than 5%.

Keywords： lithium ion battery (LIB) ; state of health ; internal resistance ; equivalent circuit ; time constant

PDF (1039KB) 元数据 多维度评价 相关文章 导出 EndNote| Ris| Bibtex  收藏本文

本文引用格式

潘斌, 董栋, 钱东培, 钮树强, 刘双宇, 姜银珠. 磷酸铁锂电池内阻分量快速检测方法. 浙江大学学报(工学版)[J], 2021, 55(1): 189-194 doi:10.3785/j.issn.1008-973X.2021.01.022

PAN Bin, DONG Dong, QIAN Dong-pei, NIU Shu-qiang, LIU Shuang-yu, JIANG Yin-zhu. Quick identification of internal resistance components for lithium ion battery with LiFePO4 cathode . Journal of Zhejiang University(Engineering Science)[J], 2021, 55(1): 189-194 doi:10.3785/j.issn.1008-973X.2021.01.022

为了保证电池系统稳定、可靠运行，对锂离子电池的健康状态（state of health，SOH）进行准确评估是电池运维环节的重点之一[1]. 锂离子电池内阻对应电池内部的电化学过程，是表征电池健康状态的关键参数[2-3]，主要包括欧姆内阻和极化内阻[4]. 其中欧姆内阻Ro包含电极材料、集流体等电池零部件的接触电阻以及电解液、隔膜电阻，在充放电过程中一般保持稳定. 极化内阻被认为由界面电荷转移引起的电化学极化内阻Rct和浓差极化内阻Rd组成. 研究各内阻分量的检测和参数辨识方法，对于分析电池健康状态具有重要意义.

电化学交流阻抗谱（electrochemical impedance spectroscopy，EIS）是较常用的表征电池电化学性能的分析方法[3-5]，可以测试电池的欧姆内阻、极化内阻及扩散内阻信息. EIS测试受频率响应速度的限制，通常测试时间较长[6]，同时EIS检测设备复杂且昂贵[7]，不适合于现场快速无损检测. 基于交流注入法的电池内阻测试仪因精度高、操作简单，得到广泛应用；测试仪通过测试电池端电压响应计算Ro[8]，由于输出信号的频率高、振幅小，可以忽略该过程中的极化效应. 交流内阻测试只能得到欧姆内阻，无法测试极化内阻分量.

直流脉冲测试是常用的电池直流内阻（direct current resistance，DCR）测试方法，原理是对处于稳态的电池进行短时间的充电或放电后静置，分析该过程中电池的电压变化，进而计算电池内阻[9-11]. 由于电池内部界面电荷转移速度非常快，在实际测量过程中，很难将电荷转移内阻与欧姆内阻分离开[12]. 超短的采样时间可以避免电化学极化的影响，但会造成严重的数据冗余，并降低系统可靠性[13]. 采用数值模拟方法间接计算电池极化内阻，得到研究人员的广泛关注[6, 14]，但这些研究中都没有考虑电化学极化过程时间常数，限制了辨识方法的适用性.

考虑到电池中锂离子扩散过程远慢于界面电荷转移过程[15]，一般DCR测试采样时间远大于界面电荷转移时间，可以认为DCR测试中瞬时电压变化对应的电阻R1主要由欧姆内阻和电化学极化内阻组成. 基于该假设，本文通过EIS、DCR以及交流内阻测试，分析电池各内阻分量的特性. 以磷酸铁锂电池为例，研究锂离子电池内阻分量的无损检测方法. 在分析各内阻分量对应的时间常数范围的基础上，提出直流脉冲测试结合交流内阻测试的内阻分量检测辨识方法. 本文提出的辨识方法简单易用，可靠性较高且可以实现在线测量，为评估储能电池系统SOH提供了支持.

1. 实验过程

使用额定容量C为1 500 mA·h的商用18650磷酸铁锂电池为研究对象，进行循环老化测试. 电池测试使用深圳新威BTS-5V3A测试仪，测试在常温条件（25 °C）下开展，最大电压采样频率为10 Hz. 为了获得电池实际最大容量，采用恒流-恒压充电方法，将电池充电至荷电状态（state of charge，SOC）为100%，以C/25电流放电至截止电压，记录此时的容量，将此时的容量作为电池实际可用容量.

采用直流脉冲法，结合交流内阻测试仪测试电池内阻分量. 具体包括以下步骤.

1）以1 C倍率对电池进行短时间放电，使得放电完毕后电池SOC为10%~90%，之后静置15 min. 图1中，V为电池端电压，I为电流。如图1所示，t1时刻前电池脉冲放电，此时电池SOC调整至设定值，t1至t2时间段的15 min停止放电，电池静置.

图 1

新窗口打开| 下载原图ZIP| 生成PPT

图 1   直流脉冲测试电流及电压信号示意图

Fig.1   Schematic of current and voltage profile in direct current pulse


2）记录电池最后一次静置初始时刻的瞬时电压跃变ΔU1及缓慢电压变化值ΔU2. 电池的直流内阻R1和R2为

$ {R_1} = \Delta {U_1}/\Delta I, $

$ {R_2} = \Delta {U_2}/\Delta I. $

3）使用交流电池内阻测试仪（RC 3562）对静置后的电池进行交流内阻测试，记为Ro，表征电池欧姆内阻. 该设备通过测试交流电压响应自动计算内阻，测试精度为0.5%，最小电阻分辨率为1 μΩ，交流测试信号为频率为1 kHz的5 mA电流.

在电池稳定后，使用IVIUM VERTEX电化学工作站进行电池EIS测试，仪器的最小电流分辨率为15 pA，电压分辨率为0.4 μV. 测试频率为0.05~1000 Hz，交流电压振幅为5 mV. 使用Zview 软件拟合测试得到Nyquist图，获得欧姆内阻和极化内阻.

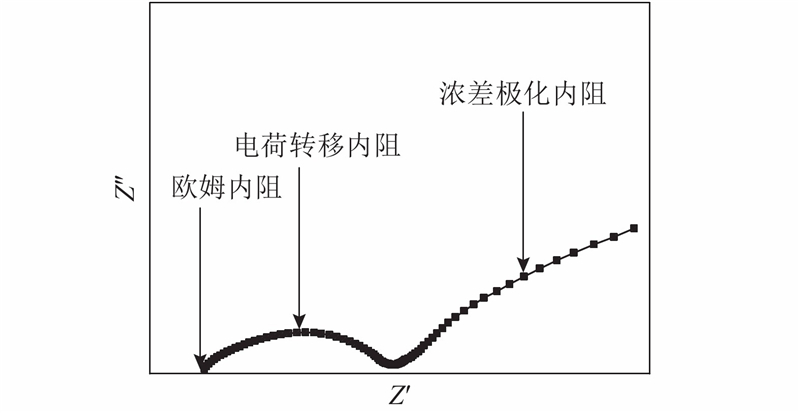
锂离子电池电化学阻抗谱与各内阻分量的对应关系如图2所示.

图 2

新窗口打开| 下载原图ZIP| 生成PPT

图 2   锂离子电池电化学阻抗谱与内阻分量的对应关系

Fig.2   Corresponding relationship between EIS and internal resistance of lithium ion battery


2. 结果与讨论

2.1. 界面电荷转移电阻

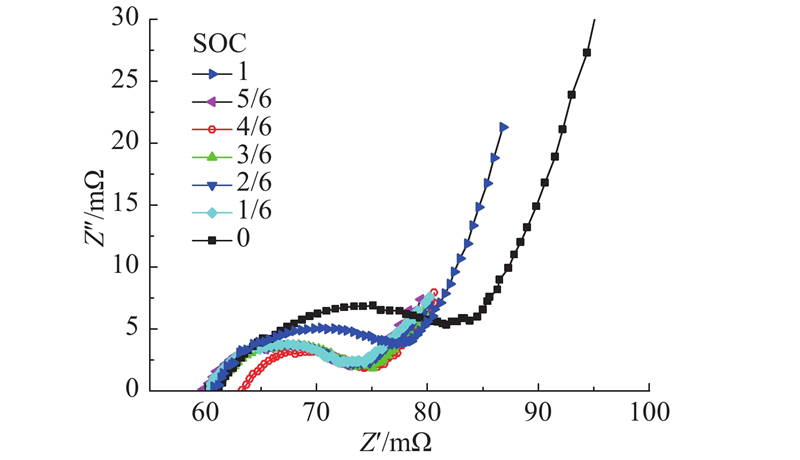
EIS测试结果如图3所示，当电池SOC为1/6~5/6时，电化学极化内阻与SOC的关系不大.

图 3

新窗口打开| 下载原图ZIP| 生成PPT

图 3   不同荷电状态下电池电化学阻抗测试结果

Fig.3   EIS of cell with different SOC


如表1所示为不同SOC下的电池EIS拟合值。表中，Rct与Cct分别为电荷转移内阻和双电层等效电容，τ为RC环节时间常数. 电化学极化反映锂离子在电极活性材料界面转移的过程。该过程的速度很快，当电池不处于满充或者满放状态时，界面电荷转移阻抗可以认为近似相同[16].

表 1   不同荷电状态下电池电化学阻抗参数拟合结果

Tab.1  EIS parameters of cell with different SOC

SOC Ro /mΩ Cct /F Rct /mΩ τ /ms
1 60.09 2.68 20.69 55.45
5/6 59.77 1.51 14.00 21.07
4/6 63.35 1.49 11.50 17.08
3/6 60.82 1.38 13.34 18.40
2/6 60.28 1.38 13.47 18.55
1/6 60.36 1.23 12.67 15.63
0 60.60 2.58 26.70 68.88

新窗口打开| 下载CSV


界面电荷转移过程可以用Bulter-Volmer方程[6, 14]描述：

$ I = A {i_0}\left[\exp\; \left( {\frac{{\alpha F}}{{RT}}\eta } \right) - \exp \; \left( - \frac{{(1 - \alpha )F}}{{RT}}\eta \right)\right]. $

式中：F、R、T分别为法拉第系数、理想气体常数和绝对温度；A为界面面积；i0为交换电流密度；η为反应过电位；α为传递系数，通常保持稳定，此处α=0.5[14]. 式（3）可以写为

$ I = {I_0}[\exp\; \left( {\eta /0.052} \right) - \exp\; ( - \eta /0.052)]. $

式中：I0为界面交换电流. 由式（4）可得电荷转移过程中界面双电层的电流和电压的关系，如图4所示，其中交换电流与电池极片面积有关. 当电压小于阈值时，电流与电压基本线性相关. 考虑到Rct很小，对于1 C倍率充放电的使用条件，双电层上电压通常不会大于100 mV，由电阻计算公式

图 4

新窗口打开| 下载原图ZIP| 生成PPT

图 4   界面电荷转移过程中的电流变化

Fig.4   Current change in charge transfer process


$ {R_{{\rm{ct}}}} = \frac{{{\rm{d}}\eta }}{{{\rm{d}}I}} $

可知，当过电位很小时， ${R_{{\rm{ct}}}} \propto i_0^{ - 1}$，Rct只与平均交换电流密度有关. 在一定SOC范围内，当正、负极界面的活性不变时，可以认为Rct不变化，这与文献[17]相符.

当SOC=0时，磷酸铁锂电极由于持续嵌锂，活性材料内可嵌锂空位减少至一定程度引起界面反应变慢，此时锂离子嵌入会受到已占锂离子库仑斥力，导致交换电流减小，从而表现为Rct突然增大. 当SOC=100%时，石墨负极由于嵌锂率较高，Rct会显著增大[15].

锂离子电池等效电路模型可以由图5表示. 在稳态情况下，表征电化学极化阻抗的Rct及Cct二者两端的电压相等. 对于RC环节，当电池由恒流放电转为静置状态时，电压变化为

图 5

新窗口打开| 下载原图ZIP| 生成PPT

图 5   锂离子电池等效电路模型

Fig.5   Equivalent circuit model of lithium ion battery


$ {U_{\rm{p}}} = I{R}_{{\rm{ct}}} \exp\; \left( - \frac{{t - {t_0}}}{\tau }\right). $

式中：

$ \tau = {R_{{\rm{ct}}}}{C_{{\rm{ct}}}}, $

Up为Rct两端电压. 由EIS测试结果可以看出，在一定的SOC范围内，τ保持为15~20 ms（见表1），远小于采样时间间隔. 由式（6）可知，当静置阶段电压采样开始时，Up已经衰减至可忽略不计，故R1可以认为是欧姆内阻和电荷转移内阻之和.

2.2. 浓差极化内阻分析

直流脉冲测试及交流内阻测试仪测试结果如图6所示. 图中，Rint为使用交流内阻测试仪测得的电池欧姆内阻. R1大于Rint，且在SOC工作范围内基本不变，说明电池欧姆内阻和界面电荷转移内阻相对保持稳定. 在该SOC区间内，电池正负极与电解液界面的电化学活性总体变化较小. R2随着SOC的增加上下波动，并出现明显峰值，表明浓差极化内阻不仅受电池内锂离子浓度分布的影响，而且与电极活性材料嵌锂率有关，这可能是由于电极材料发生相变过程导致的[18].

图 6

新窗口打开| 下载原图ZIP| 生成PPT

图 6   不同荷电状态下直流内阻测试结果

Fig.6   Measurement results of DCR at different SOC


通常采用RC环节模拟电池浓差极化过程，即图1中最大值为U2的缓慢回复电压[10]。

$ V = {V_{{\rm{oc}}}} - I{R_{\rm{d}}} \exp\; \left( - \frac{{t - {t_0}}}{\tau }\right). $

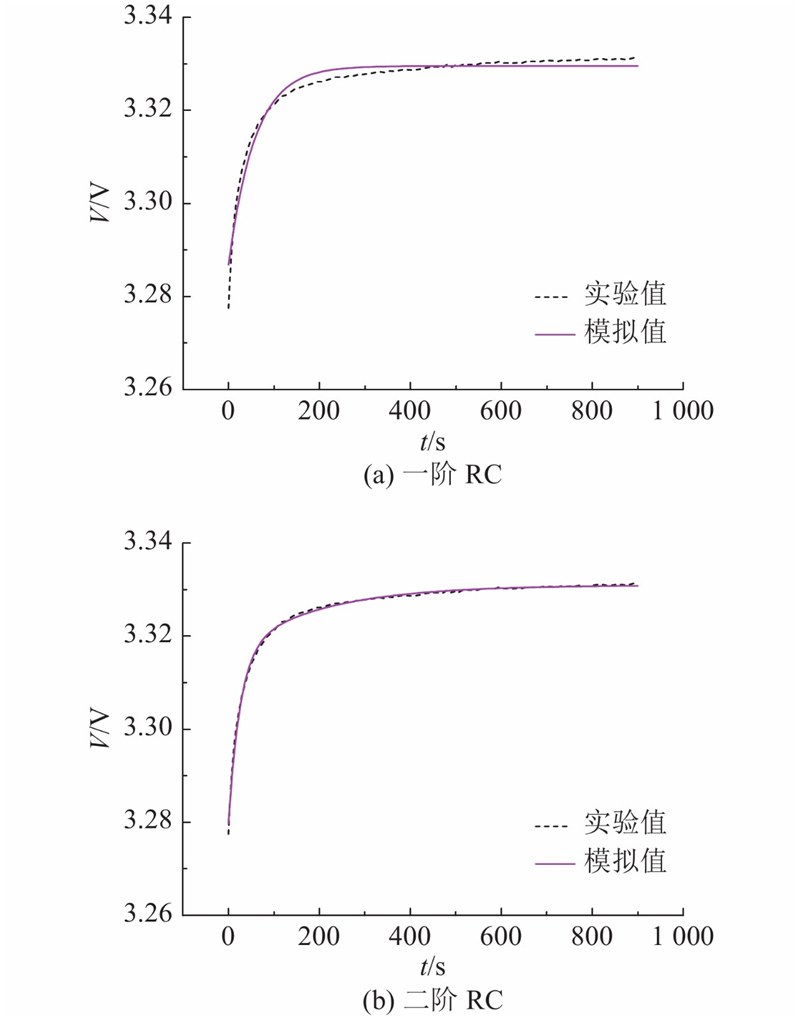
由于电池正极、负极活性材料的扩散系数不同，往往单RC环节不能很好地拟合电压释放曲线[19]. 采用双RC环节等效元件模拟，将浓差极化内阻及等效电容Rd/Cd表示为由Rd1/Cd1与Rd2/Cd2串联，

$ V = {V_{{\rm{oc}}}} - I{R_{{\rm{d}}1}} \exp \; \left( - \frac{{t - {t_0}}}{{{\tau _1}}}\right) - I{R_{{\rm{d}}2}} \exp\; \left( - \frac{{t - {t_0}}}{{{\tau _2}}}\right). $

式（8）、（9）的模拟结果如图7（a）、（b）所示，双RC环节模型显示了更高的精度，这与报道结果相符[7, 20].

图 7

新窗口打开| 下载原图ZIP| 生成PPT

图 7   直流内阻测试中电压释放阶段模拟结果

Fig.7   Fitting results of voltage relaxation process in DCR test


二阶RC等效模型计算结果如表2所示. 由于电池内不同材料组分中的锂离子扩散过程具有一定差异[6]，2个不同的RC环节参数计算结果差别较大，Rd随SOC变化的主要贡献源自具有较大时间常数的内阻分量. 由模拟结果可以发现，2个浓差极化分量的时间常数均远大于测试系统采样时间和电化学极化时间常数，说明R2为浓差极化内阻，基本不存在界面电荷转移内阻分量.

表 2   采用二阶等效电路时的浓差极化内阻模拟计算结果

Tab.2  Calculation results of concentration polarization resistance by second order RC models

SOC τ1 /s τ2 /s Rd1 /mΩ Rd2 /mΩ Rd /mΩ
1/6 25.18 193.52 24.40 9.74 34.14
2/6 30.97 921.05 23.99 15.12 39.11
3/6 22.74 183.15 21.97 9.66 31.63
4/6 21.86 256.04 25.36 12.21 37.57
5/6 29.12 301.70 31.86 20.68 52.54

新窗口打开| 下载CSV


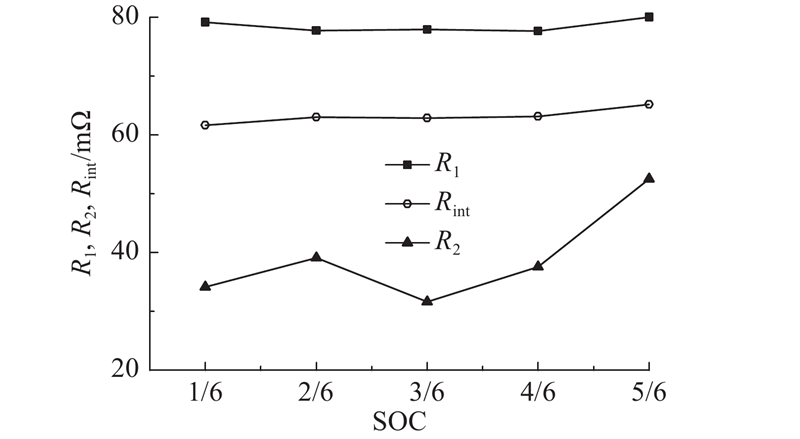
2.3. 电池内阻分量计算

交流测试仪输出1 kHz的小电流信号，通过测量电压响应，经过锁相放大器滤除噪声后，获得内阻. 由于信号频率高且强度很小，可以忽略测试过程中的极化效应，测量值可以认为是电池欧姆内阻分量. 不同SOC下交流内阻测试值如图8所示. 结果显示了较高的精度，与EIS欧姆内阻测试结果比较，平均误差为3.7%.

考虑到直流脉冲测试R1包含了欧姆内阻和电化学极化内阻，有

$ {R_{{\rm{ct}}}} = {R_1} - {R_{{\rm{o}}.}} $

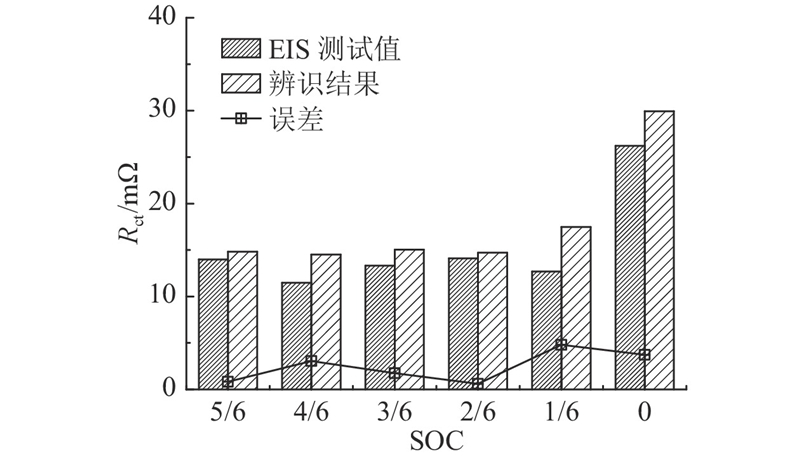
DCR测试电流为1.5 A，双电层上电压Up仅约为20 mV. 由式（3）~（5）可知，Rct可以认为与测试电流无关. 如图9所示为Rct辨识结果与EIS测试值的对比，两者保持了较高的一致性，Rct误差为0.61~4.80 mΩ，最小误差不大于5%. 当SOC=0时，尽管Rct明显增大，计算结果仍较准确，显示了辨识方法较高的可靠性.

图 8

新窗口打开| 下载原图ZIP| 生成PPT

图 8   交流内阻测试仪与电化学阻抗测得电池欧姆内阻

Fig.8   Ohmic resistance obtained by AC resistance tester and EIS


图 9

新窗口打开| 下载原图ZIP| 生成PPT

图 9   电池电化学极化内阻辨识结果

Fig.9   Activation polarization resistance identified by proposed method


3. 结　语

锂离子电池内阻是表征健康状态的重要参数，本文通过EIS、DCR及交流内阻测试分析电池各内阻分量的特性，以磷酸铁锂电池为例，研究锂离子电池内阻分量的无损检测方法.

电池欧姆内阻不受SOC和测试电流的影响，但在SOC接近100%或0时，由于电荷转移困难，Rct显著增大. Bulter-Volmer方程的模拟结果表明，在电池正常工作电流范围内，Rct基本不随电流变化，可以认为界面电荷转移过程时间常数始终为15~20 ms，远小于DCR采样周期. 由于电池正负极内锂离子扩散的差异，浓差极化过程表示为二阶RC环节能够获得更高的精度.

根据RC环节零输入响应状态下的极化电压计算公式可知，在DCR测试得到的瞬时响应内阻R1包含欧姆内阻分量和电化学极化内阻分量，可以通过R1与交流内阻测试得到的欧姆内阻作差，得到电化学极化内阻. 使用该方法得到的辨识结果显示较好的准确性.

电池内阻分量分别对应了电池各组分的健康状态[4]，欧姆内阻增加表征电池电接触衰减（CL），电池电化学极化内阻增加受可用锂离子损失（LLI）的影响，浓差极化内阻增加与电极活性材料损失（LAM）密切相关. 采用提出的内阻分量辨识方法，通过测试不同循环次数后电池的内阻分量，可以有效评价SOH，为电池系统的稳定可靠运行提供重要支持.

参考文献

BERECIBAR M

Accurate predictions of lithium-ion battery life

[J]. Nature, 2019, 568 (7752): 325- 326

DOI:10.1038/d41586-019-01138-1      [本文引用: 1]

SAFARI M, DELACOURT C

Aging of a commercial Graphite/LiFePO4 cell

[J]. Journal of the Electrochemical Society, 2011, 158 (10): A1123- A1135

DOI:10.1149/1.3614529      [本文引用: 1]

ANDRE D, MEILER M, STEINER K, et al

Characterization of high-power lithium-ion batteries by electrochemical impedance spectroscopy. I. Experimental investigation

[J]. Journal of Power Source, 2011, 196 (12): 5334- 5341

DOI:10.1016/j.jpowsour.2010.12.102      [本文引用: 2]

PASTOR-FERNANDEZ C, UDDIN K, CHOUCHELAMANE G H, et al

A comparison between electrochemical impedance spectroscopy and incremental capacity-differential voltage as Li-ion diagnostic techniques to identify and quantify the effects of degradation modes within battery management systems

[J]. Journal of Power Source, 2017, 360: 301- 318

DOI:10.1016/j.jpowsour.2017.03.042      [本文引用: 2]

SURESH P, SHUKLA A K, MUNICHANDRAIAH N

Temperature dependence studies of a. c. impedance of lithium-ion cells

[J]. Journal of Applied Electrochemistry, 2002, 32 (3): 267- 273

DOI:10.1023/A:1015565404343      [本文引用: 1]

CUI Y, ZUO P, DU C, et al

State of health diagnosis model for lithium ion batteries based on real-time impedance and open circuit voltage parameters identification method

[J]. Energy, 2018, 144: 647- 656

DOI:10.1016/j.energy.2017.12.033      [本文引用: 4]

QIAN K, HUANG B, RAN A, et al

State-of-health (SOH) evaluation on lithium-ion battery by simulating the voltage relaxation curves

[J]. Electrochimica Acta, 2019, 303: 183- 191

DOI:10.1016/j.electacta.2019.02.055      [本文引用: 2]

江海, 李革臣, 苏淇桂, 等

基于MSP 430单片机的便携式电池内阻测试仪

[J]. 电源技术, 2007, 31 (7): 548- 550

DOI:10.3969/j.issn.1002-087X.2007.07.012      [本文引用: 1]

JIANG Hai, LI Ge-chen, SU Qi-gui, et al

Portable instrument based on MSP 430 MCU for testing battery internal impedance

[J]. Chinese Journal of Power Source, 2007, 31 (7): 548- 550

DOI:10.3969/j.issn.1002-087X.2007.07.012      [本文引用: 1]

XIONG R, LI L L, TIAN J P

Towards a smarter battery management system: a critical review on battery state of health monitoring methods

[J]. Journal of Power Source, 2018, 405: 18- 29

DOI:10.1016/j.jpowsour.2018.10.019      [本文引用: 1]

ZOU Y, HU X, MA H, et al

Combined state of charge and state of health estimation over lithium-ion battery cell cycle lifespan for electric vehicles

[J]. Journal of Power Source, 2015, 273: 793- 803

DOI:10.1016/j.jpowsour.2014.09.146      [本文引用: 1]

JIANG Y, JIANG J C, ZHANG C P, et al

State of health estimation of second-life LiFePO4 batteries for energy storage applications

[J]. Journal of Cleaner Production, 2018, 205: 754- 762

DOI:10.1016/j.jclepro.2018.09.149      [本文引用: 1]

REMMLINGER J, BUCHHOZ M, MEILERM, et al

State-of-health monitoring of lithium-ion batteries in electric vehicles by on-board internal resistance estimation

[J]. Journal of Power Source, 2011, 196: 5357- 5363

DOI:10.1016/j.jpowsour.2010.08.035      [本文引用: 1]

徐升荣, 曾洁, 张育华, 等

基于脉冲电流测量锂电池内阻的实验平台设计

[J]. 电子测量技术, 2018, 41 (1): 69- 73

[本文引用: 1]

XU Sheng-rong, ZENG Jie, ZHANG Yu-hua, et al

Design of experimental platform for measuring internal resistance of lithium batteries based on pulse current

[J]. Electronic Measurement Technology, 2018, 41 (1): 69- 73

[本文引用: 1]

LIU S, JIANG J, SHI W, et al

Butler–Volmer-equation-based electrical model for high-power lithium Titanate batteries used in electric vehicles

[J]. IEEE Transactions on Industrial Electronics, 2015, 62 (12): 7557- 7568

DOI:10.1109/TIE.2015.2449776      [本文引用: 3]

凌仕刚, 吴娇杨, 张舒, 等

锂离子电池基础科学问题(ⅫⅠ): 电化学测量方法

[J]. 储能科学与技术, 2015, 4 (1): 83- 103

DOI:10.3969/j.issn.2095-4239.2015.01.010      [本文引用: 2]

LING Shi-gang, WU Jiao-yang, ZHANG Shu, et al

Fundamental scientific aspects of lithium ion batteries(Ⅻ Ⅰ): electrochmical measurement

[J]. Energy Storage Science and Technology, 2015, 4 (1): 83- 103

DOI:10.3969/j.issn.2095-4239.2015.01.010      [本文引用: 2]

张彩萍, 姜久春, 张维戈, 等

梯次利用锂离子电池电化学阻抗模型及特性参数分析

[J]. 电力系统自动化, 2013, 37 (1): 54- 58

DOI:10.7500/AEPS201210170      [本文引用: 1]

ZHANG Cai-ping, JIANG Jiu-chun, ZHANG Wei-ge, et al

Characterization of electromical impedence model and parameters for Li-ion batteries echelon use

[J]. Automation of Electric Power Systems, 2013, 37 (1): 54- 58

DOI:10.7500/AEPS201210170      [本文引用: 1]

徐晶, 张彩萍, 汪国秀, 等

梯次利用锂离子电池欧姆内阻测试方法研究

[J]. 电源技术, 2015, 39 (2): 252- 256

DOI:10.3969/j.issn.1002-087X.2015.02.009      [本文引用: 1]

XU Jing, ZHANG Cai-ping, WANG Guo-xiu, et al

Research on testing method of ohmic resistance for Li-ion batteries echelon use

[J]. Chinese Journal of Power Source, 2015, 39 (2): 252- 256

DOI:10.3969/j.issn.1002-087X.2015.02.009      [本文引用: 1]

DUBARRY M, LIAW B Y

Development of a universal modeling tool for rechargeable lithium batteries

[J]. Journal of Power Source, 2007, 174 (2): 856- 860

DOI:10.1016/j.jpowsour.2007.06.157      [本文引用: 1]

LEI P, WANG T, RENGUI LU, et al

Development of a voltage relaxation model for rapid open-circuit voltage prediction in lithium-ion batteries

[J]. Journal of Power Source, 2014, 253: 412- 418

DOI:10.1016/j.jpowsour.2013.12.083      [本文引用: 1]

WANG Q Q, KANG J Q, TAN Z X, et al

An online method to simultaneously identify the parameters and estimate states for lithium ion batteries

[J]. Electrochimica Acta, 2018, 289: 376- 388

DOI:10.1016/j.electacta.2018.08.076      [本文引用: 1]

/

〈 〉