2. 无锡透平叶片有限公司, 江苏 无锡 214023
2. Wuxi Turbine Blade Co., Ltd., Wuxi 214023, China
近年来,模具精锻技术得到快速发展,据统计国外80%的航空叶片是通过模具精锻技术制造的[1-2]。与国外相比,我国航空叶片精锻模具设计技术较为落后,在国内,设计一套模具要花费1~2周,而国外只需花费2~3 d,且叶片种类繁多、批量小、形状复杂、精度要求高,还会加重叶片精锻模具设计的任务[3]。在锻压过程中,由于模具的设计参数对叶片的成形规律和模具寿命有着重要影响,不合理的模具设计参数会导致模具磨损加剧,影响模具寿命[4-6]。因此,研究如何提高叶片精锻模具设计效率、优化模具设计参数、缩短产品开发周期、延长模具使用寿命,对提高企业经济效益和核心竞争力具有重要意义[7]。
本文基于UG/Open API开发了模具CAD系统,实现叶片模具的参数化设计;基于Archard修正理论,借助Deform-3D软件对不同设计参数下叶片模具进行仿真模拟,通过正交试验分析锻压速度、成形角度、桥部厚度对叶片模具磨损与寿命的影响,得到最优工艺组合参数,并对该组合参数的设计规则进行优化;最后通过试验对优化后模具的磨损和寿命进行了分析验证。
1 叶片模具参数化设计为提高航空叶片模具设计效率,江南大学机械工程学院与某企业合作,运用UG二次开发技术开发了模具CAD系统,为叶片模具设计定制专用模板,如图 1所示。

|
| 图 1 航空叶片模具设计菜单界面 Fig.1 Aviation blade die design menu interface |
在锻压成形过程中,Archard理论是计算模具磨损的常用模型,在该理论模型中,模具材料硬度和磨损系数为定值。而在实际热锻成形过程中,模具温度随锻压时间发生变化,材料的硬度与磨损系数随温度变化而变化,故材料的硬度和磨损系数应为变量。Lee和Behrens等[8-9]对不同锻压温度下的模具(材料为钢H13)进行了摩擦磨损试验,得出了模具温度与材料硬度和磨损系数之间的关系,提出了Archard的修正模型:
| $ \begin{array}{l} W = f\left( T \right)\frac{1}{{H\left( T \right)}}\int {pv{\rm{d}}\mathit{t}} \\ f\left( T \right) = \left[{29.29 \times \ln \;T-168.73} \right] \times {10^{ - 6}}\\ H\left( T \right) = 9\;216.4 \times {T^{ - 0.505}} \end{array} $ |
式中:f(T)为材料磨损系数;H(T)为材料硬度;W为磨损量;p为锻造压力;v为材料流动速度;T为模具温度。
在锻压成形过程中,模具型腔不同部位的温度、压力、材料流动速度等随时间变化而变化[10-11],故第i处第j时间段的磨损量可表示为:
| $ \Delta {w_{ij}} = {f_{ij}}\left( T \right)\frac{1}{{{H_{ij}}\left( T \right)}}{p_{ij}}{v_{ij}}\Delta {t_j} $ |
总磨损量为:
| $ {W_i} = \int_0^n {{f_{ij}}\left( T \right)} \frac{{{p_{ij}}{v_{ij}}}}{{{H_{ij}}\left( T \right)}}{\rm{d}}\mathit{t} $ |
式中:pij为模具型腔i处第j时间段的压力;vij为模具型腔i处第j时间段的材料流动速度;n为锻压次数。
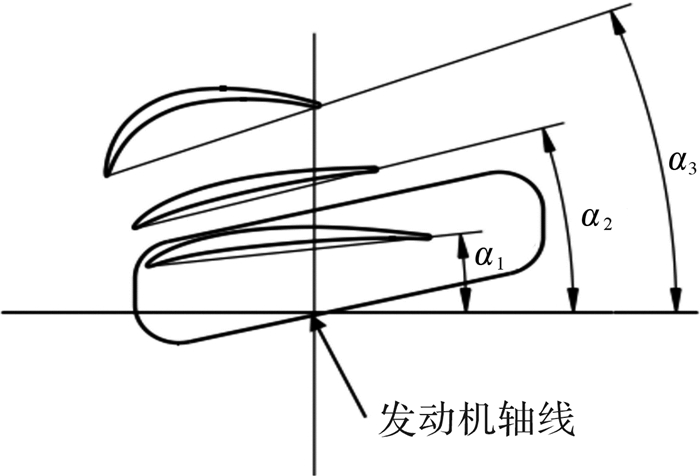
2.2 基于正交试验的Deform-3D仿真分析成形角是指叶片模具截面弦长线与发动机轴线夹角,如图 2所示。桥部、仓部结构如图 3所示。采用正交试验作为参数选优的方法[12-13],以锻压速度(A)、成形角度(B)、桥部厚度(C)三个因素为变量,借助正交试验方法对某叶片成形过程进行仿真,研究锻压成形时不同因素水平对成形载荷、最大成形应力、横向错模力和模具磨损量的影响。因素水平表如表 1所示,正交试验方案如表 2所示。
|
| 图 2 叶片成形角度示意图 Fig.2 Blade forming angle diagram |

|
| d—桥部厚度;D—仓部厚度;L—桥部宽度 图 3 模具桥部和仓部结构示意图 Fig.3 Structure diagram of bridge and warehouse of die |
| 水平 | 因素 | ||
| 锻压速度/(mm/s) | 成形角度/(°) | 桥部厚度1)/mm | |
| 1 | 15 | 12 | 0.8h |
| 2 | 25 | 15 | 1.1h |
| 3 | 35 | 17 | 1.4h |
| 注:1)为进出气边厚度。 | |||
| 序号 | 水平 | 成形载荷/kN | 最大成形应力/MPa | 横向错模力/kN | 模具磨损量/mm | ||
| 锻压速度(A) | 成形角度(B) | 桥部厚度(C) | |||||
| 1 | 1 | 1 | 1 | 6 710 | 2 440 | 737 | 0.000 550 |
| 2 | 1 | 2 | 2 | 6 130 | 2 190 | 285 | 0.000 220 |
| 3 | 1 | 3 | 3 | 6 010 | 2 080 | 577 | 0.000 412 |
| 4 | 2 | 1 | 2 | 5 210 | 1 910 | 512 | 0.000 396 |
| 5 | 2 | 2 | 3 | 5 360 | 1 750 | 274 | 0.000 215 |
| 6 | 2 | 3 | 1 | 5 530 | 2 020 | 543 | 0.000 408 |
| 7 | 3 | 1 | 3 | 6 110 | 2 060 | 596 | 0.000 418 |
| 8 | 3 | 2 | 1 | 6 370 | 2 240 | 340 | 0.000 243 |
| 9 | 3 | 3 | 2 | 6 190 | 2 090 | 587 | 0.000 417 |
在进行Deform-3D仿真时,需要设置对象的属性和边界条件[14-16]:
1) 锻坯材料:Ti-6Al-4V,设为塑性体,终锻温度为950 ℃,网格数量为50 388个。
2) 模具材料:4Cr5MoSiV1,设为刚体;终锻时,上模参考温度设为250 ℃,网格数量为147 212个,运动方向为Y轴方向;下模固定,参考温度为300 ℃,网格数量为154 300个;硬度为55HRC。
3) 接触关系:摩擦因子设为0.3;热传导系数设为11;磨损模型为Archard模型。
4) 模拟控制:总行程约为24 mm, 步数设置100步,步长为0.25 mm。
借助Deform-3D软件对表 2中的9种参数设计方案进行仿真模拟,得到不同参数组合下的成形载荷、最大成形应力、横向错模力和模具磨损量,并计算得到偏差量总和,如表 3。为综合考评各水平、各因素对研究对象的影响,需对得到的试验数据进行直观分析和方差分析。
| 研究对象 | 偏差量总和1) | |||
| 成形载荷 | K1j | 18 850 | 18 030 | 18 610 |
| K2j | 16 100 | 17 860 | 17 530 | |
| K3j | 18 670 | 17 730 | 17 480 | |
| 最大成形应力 | K1j | 6 710 | 6 410 | 6 700 |
| K2j | 5 680 | 6 180 | 6 190 | |
| K3j | 6 390 | 6 190 | 5 890 | |
| 横向错模力 | K1j | 1 599 | 1 845 | 1 620 |
| K2j | 1 329 | 899 | 1 384 | |
| K3j | 1 523 | 1 707 | 1 447 | |
| 模具磨损量 | K1j | 1 266 | 1 364 | 1 231 |
| K2j | 1 045 | 758 | 1 057 | |
| K3j | 1 108 | 1 237 | 1 071 | |
| 注:1)Kij(i=1, 2, 3)表示各水平因素偏差量总和。 | ||||
根据直观分析可得出9种设计方案与研究对象之间的关系,如图 4所示。根据图 4可得出最佳因素水平组合:就成形载荷而言,最佳组合为A2B3C3;就最大成形应力而言,最佳组合为A2B2C3;就横向错模力而言,最佳组合为A2B2C2;就模具磨损量而言,最佳组合为A2B2C2。

|
| 图 4 不同因素水平下的仿真值 Fig.4 Simulation values at different levels of factors |
根据方差分析得出各因素与研究对象之间的显著性关系,如表 4所示。对于成形载荷,因素A表现为显著,B,C不显著;对于最大成形应力,因素A,C表现为高度显著,B显著;对于横向错模力,因素B表现为显著,A,C不显著;对于模具磨损量,因素B表现为显著,A,C不显著。
| 方差来源 | 偏差平方和 | F值(显著性)1) | |||||||
| 锻造载荷 | 最大成形应力 | 横向错模力 | 磨损量 | 锻造载荷 | 最大成形应力 | 横向错模力 | 磨损量 | ||
| A | 15 777 756 | 185 267 | 12 924 | 0.000 000 4 | 19.68* | 397.00** | 1.98 | 1.15 | |
| B | 15 089 | 11 267 | 174 092 | 0.000 006 8 | 0.19 | 24.14* | 26.62* | 22.40* | |
| C | 271 756 | 111 800 | 9 955 | 0.000 000 6 | 3.39 | 239.57** | 1.52 | 1.48 | |
| e2) | 80 156 | 467 | 6 540 | 0.000 000 4 | |||||
| F3) | F0.012, 2=99;F0.052, 2=19;F0.12, 2=9;F0.252, 2=3 | ||||||||
| 注:1)**表示高度显著;*表示显著; 2)e为误差项; 3)F为方差比。 | |||||||||
通过以上分析可知各因素与研究对象之间的关系和显著性。就成形载荷而言,因素A是主要影响因素,故选择A2;就最大成形应力而言,因素A,B,C的影响都很大;就横向错模力和模具磨损量而言,因素B是主要影响因素,故选择B2。因此最佳因素水平组合为A2B2C3。但考虑到叶片锻压成形时的实际成形规律,应确定最佳组合为A2B2C2。
3 模具设计参数优化 3.1 成形角度优化根据上述分析知,成形角度对模具的横向错模力与磨损量的影响显著,不合理的成形角度会引起模具侧向力增加,导致其横向移位,使模具磨损加剧,严重缩短了模具的使用寿命。原有成形角度设计方法复杂且误差较大,影响了模具的设计效率与精度[17],通过多次仿真模拟和工程试验,分析得出了叶片结构与成形角度之间的关系,即三截面角度平均法:
| $ {\alpha _0} = \frac{{{\alpha _1} + {\alpha _2} + {\alpha _3}}}{3} $ |
式中:α1为叶尖某截面弦长线与发动机轴线夹角;α2为叶身某截面弦长线与发动机轴线夹角;α3为叶根某截面弦长线与发动机轴线夹角。
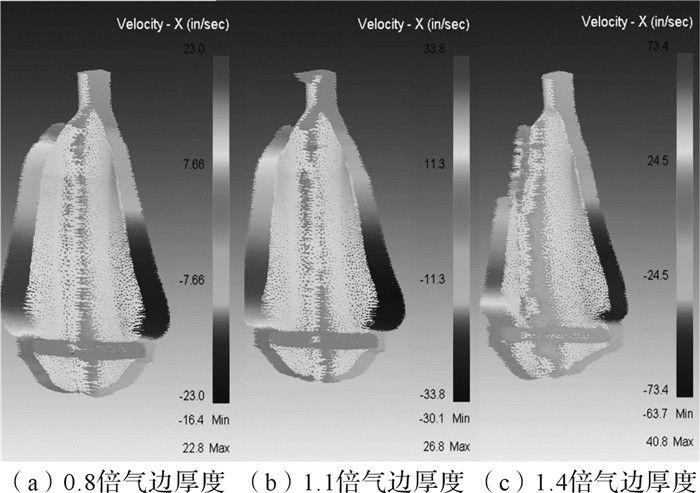
3.2 桥部厚度优化根据仿真和工程试验分析可知,叶片模具桥部厚度对叶片成形规律有着显著的影响。由于叶片进出气边厚度较薄,为使进出气边充型完整,就必须减小桥部厚度以增加金属的流动阻力,但过小的桥部厚度会增加模具成形载荷,而桥部厚度过大会导致叶片成形规律难以控制[18]。因此为了既能保证金属充型完整又能让其顺畅流出模具,桥部厚度的合理设计变得很重要。图 5为不同桥部厚度下的叶片终锻成形图,由图可知:过小的桥部厚度降低了材料的流动性,增加了成形载荷,如图 5(a);过大的桥部厚度使得叶片成形规律难以把握, 如图 5(b)。
|
| 图 5 Simulation values at different levels of factors Fig.5 Blade final forging forming map under different bridge thickness |
根据热锻过程中材料的流动特性和流体热力学理论[19],通过多次仿真和工程试验,分析得出了叶片进出气边厚度与桥部厚度、仓部厚度之间的关系,如表 5所示。
| 参数 | 设计值 | 优选值 |
| 桥部厚度d | 1.1倍进出气边厚度 | 0.6,0.8 1.0,2.4 |
| 仓部厚度D | 2~2.2倍进出气边厚度 | 1,2,2.5,3,4 |
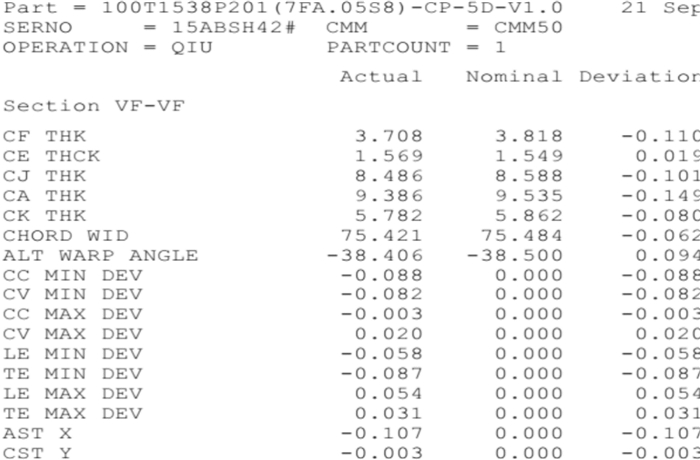
对选用最佳组合设计参数的某型号叶片模具的磨损量进行仿真模拟和试验验证,模具实体如图 6所示,在锻压过程中,模具最大允许磨损量为0.2 mm,经模拟得到该模具一次锻压成形的磨损量最大值为0.146 μm,如图 7所示, 其寿命约为1 369次。通过工程试验对经1 000次锻压成形后的模具实体的磨损量进行检测,由三坐标测量报告可知:模具一次锻压成形的磨损量最大值为0.149 μm,其寿命约为1 342次。

|
| 图 6 模具实体 Fig.6 Die entity |

|
| 图 7 模具磨损 Fig.7 The die wear |
|
| 图 8 模具检测报告 Fig.8 Die test report |
1) 基于UG/Open API技术开发了叶片精锻模具CAD系统,实现了模具参数化设计,提高了模具设计的自动化程度。
2) 基于Archard修正理论和正交试验设计方法,借助Deform-3D软件对某型号模具锻压成形过程进行了仿真,通过对仿真结果的分析,得出了各工艺因素对研究对象影响的显著性,确定了最佳设计参数组合,并对成形角度的确定方法和桥部厚度的设计规则进行了优化,使得模具设计参数的确定更加精确、简单。
3) 通过对模具磨损的仿真结果和试验结果对比可知,二者具有较好的一致性。这说明借助正交试验和仿真模拟能为航空叶片精锻模具最优化设计和寿命预测提供一种可行的方法。
| [1] |
刘维伟.
航空发动机叶片关键制造技术研究进展[J]. 航空制造技术, 2016(21): 50–56.
LIU Wei-wei. Research progress on key manufacturing technology of aeroengine blades[J]. Aeronautical Manufacturing Technology, 2016(21): 50–56. |
| [2] |
刘艳.
叶片制造技术[M]. 北京: 科学出版社, 2002: 35-41.
LIU Yan. Blade manufacturing technology[M]. Beijing: Science Press, 2002: 35-41. |
| [3] | AMANO C X R S. On the development of turbo machine blade aerodynamic design system[J]. International Journal for Computational Methods in Engineering Science & Mechanics, 2009, 10(3): 186–196. |
| [4] | JHAVER S, PAUL C P, JAIN N K. Causes of failure and repairing options for dies and molds:a review[J]. Engineering Failure Analysis, 2013, 34(8): 519–535. |
| [5] | KIM D H, LEE H C, KIM B M, et al. Estimation of die service life against plastic deformation and wear during hot forging processes[J]. Journal of Materials Processing Technology, 2005, 166(3): 372–380. DOI:10.1016/j.jmatprotec.2004.07.103 |
| [6] | LEE H C, KIM B M, KIM K H. Estimation of die service life in hot forging, considering lubricants and surface treatments[J]. Proceedings of the Institution of Mechanical Engineers Part B:Engineering Manufacture, 2003, 217(7): 1011–1022. DOI:10.1243/09544050360686860 |
| [7] | HAI Yang, DING Hua-zhang, SHAN Li, et al. Research and application of collaboration between design & manufacturing for precision forged blade[J]. Advanced Materials Research, 2012, 490-495: 2534–2539. DOI:10.4028/www.scientific.net/AMR.490-495 |
| [8] | LEE R S, JOU J L. Application of numerical simulation for wear analysis of warm forging die[J]. Journal of Materials Processing Technology, 2003, 140(1/3): 43–48. |
| [9] | BEHRENS B A, BOUGUECHA A, HADIF T, et al. Numerical and experimental investigations on the service life estimation for hot-forging dies[J]. Key Engineering Materials, 2012, 504-506: 163–168. DOI:10.4028/www.scientific.net/KEM.504-506 |
| [10] | GAO Tao, YANG He, LIU Yu-li. Backward tracing simulation of precision forging process for blade based on 3D FEM[J]. Transactions of Nonferrous Metals Society of China, 2006, 16(2): 639–644. |
| [11] | JIA W P, XIN L, JING C, et al. Temperature/stress field numerical simulation of hollow blade produced by laser rapid forming[J]. Chinese Journal of Lasers, 2007, 34(9): 1308–1312. |
| [12] |
孙宪萍, 刘强强, 杨兵, 等.
基于磨损正交试验的温挤压模具优化设计[J]. 润滑与密封, 2016, 41(6): 73–76, 92.
SUN Xian-ping, LIU Qiang-qiang, YANG Bing, et al. Optimization design on warm extrusion die based on orthogonal experiments of wear[J]. Lubrication Engineering, 2016, 41(6): 73–76, 92. |
| [13] |
占亮, 李霞, 孙礼宾, 等.
基于正交试验的曲轴热锻工艺参数优化[J]. 锻压技术, 2014, 39(7): 10–13, 18.
ZHAN Liang, LI Xia, SUN Li-bin, et al. Design optimization of process parameters of crankshaft die forging based on orthogonal experiment[J]. Forging & Stamping Technology, 2014, 39(7): 10–13, 18. |
| [14] | BARIANI P F, BRUSCHI S, NERGO T D. Integrating physical and numerical simulation techniques to design the hot forging process of stainless steel turbine blades[J]. International Journal of Machine Tools and Manufacture, 2004, 44(9): 945–951. DOI:10.1016/j.ijmachtools.2004.01.020 |
| [15] |
邵燃, 徐看.
基于Deform-3D的叶片模锻模具设计研究[J]. 热加工工艺, 2014, 43(5): 148–150, 153.
SHAO Ran, XU Kan. Study on blade forging die design based on Deform-3D[J]. Hot Working Technology, 2014, 43(5): 148–150, 153. |
| [16] |
王新军, 廖高良, 朱鼎, 等.
汽轮机空心静叶缝隙抽吸性能研究[J]. 汽轮机技术, 2012, 54(3): 195–197, 214.
WANG Xin-jun, LIAO Gao-liang, ZHU Ding, et al. Numerical simulation on suction performance of steam turbine hollow stationary blade[J]. Turbine Technology, 2012, 54(3): 195–197, 214. |
| [17] |
张国新.
汽轮机大叶片模锻成形工艺[J]. 模具技术, 2004(6): 26–30.
ZHANG Guo-xin. Die forging technology of large blades of steam turbine[J]. Die and Mould Technology, 2004(6): 26–30. |
| [18] |
齐广霞, 曹娜, 史丽坤.
GH4169合金叶片终锻成形宏观场量规律数值模拟[J]. 锻压技术, 2011, 36(5): 155–162.
QI Guang-xia, CAO Na, SHI Li-kun. Laws of macro-field numerical simulation in GH4169 alloy blade final forging[J]. Forging & Stamping Technology, 2011, 36(5): 155–162. |
| [19] |
郑丹星.
流体与过程热力学[M]. 北京: 化学工业出版社, 2010: 131-138.
ZHENG Dan-xing. Fluid and process thermodynamics[M]. Beijing: Chemical Industry Press, 2010: 131-138. |



